How To Make Quad Draw Symmetry

One to i maths interventions congenital for KS4 success

Weekly online ane to i GCSE maths revision lessons now available

Learn more than

In order to access this I need to be confident with:

Properties of 2D shapes

Plotting coordinates

Straight line graphs

Parallel and perpendicular lines

Graphs of other functions

This topic is relevant for:

GCSE Maths

Symmetry

Here we volition learn about symmetry, including line and rotational symmetry properties within polygons, angle properties, and symmetry of dissimilar line graphs.

At that place are likewise symmetry worksheets based on Edexcel, AQA and OCR exam questions, along with further guidance on where to become adjacent if y'all're still stuck.

What is symmetry?

Symmetry is when a line is fatigued through a shape to make i side of the line a reflection of the other. It is a property of a 2D polygon or 3D polyhedron.

There are two different types of symmetry that you need to be enlightened of: lines of symmetry and rotational symmetry.

Although the ii sound similar, they are fundamentally different.

What is symmetry?

Lines of symmetry

The number of lines of symmetry for a shape tin can exist determined by using a ruler to visualise when the polygon tin can be divided as into two equal pieces that are a reflection of each other.

E.g.

How many lines of symmetry does a rectangle accept?

Here, the pink and yellow sections are congruent to each other and are symmetrical to each other.

The number of lines of symmetry for any rectangle (excluding a square, which is a special rectangle), is $ii$ .

Step-past-pace guide: Lines of symmetry

Rotational symmetry

The rotational symmetry of a shape is worked out using the middle of the polygon. We need to make up one's mind where the center of the polygon is using the diagonals every bit they intersect at the middle of the shape. Once nosotros know the centre, we need to rotate the polygon around its heart to make up one's mind the order of rotation.

Tracing newspaper is very useful to determine the order of rotation of a polygon because you can trace the shape and rotate the tracing around the center, not affecting the original.

East.chiliad.

What is the social club of rotational symmetry of a rectangle?

After rotating the rectangle $180^o$ , the paradigm is an exact copy of the original. After a farther rotation of $180^o$ , we become back to the original orientation.

This ways that the order of rotational symmetry for the rectangle (excluding a square, which is a special rectangle) is $2$ .

Step-past-step guide: Rotational symmetry

How to use symmetry

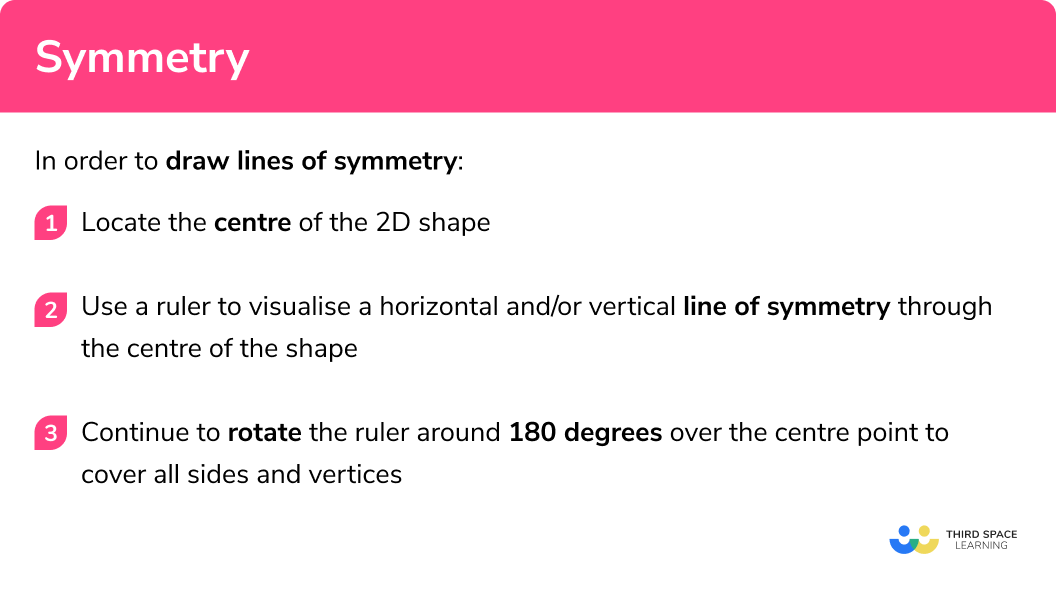
In social club to draw lines of symmetry:

Locate the centre of the 2d shape.
Use a ruler to visualise a horizontal and/or vertical line of symmetry through the centre of the shape.
Go along to rotate the ruler effectually $180$ degrees over the middle bespeak to cover all sides and vertices.

In social club to calculate the guild of rotational symmetry:

Locate the centre of the 2D shape.
Trace the shape onto a slice of tracing paper including the centre and northline.
Rotate the tracing around the eye and count the number of identical occurrences.

Explain how to apply symmetry

Explain how to use symmetry

Symmetry worksheet

Get your costless symmetry worksheet of 20+ questions and answers. Includes reasoning and practical questions.

DOWNLOAD FREE

Symmetry worksheet

Get your gratuitous symmetry worksheet of 20+ questions and answers. Includes reasoning and applied questions.

DOWNLOAD Costless

Symmetry examples

Instance i: the square (lines of symmetry)

Draw all of the lines of symmetry for the square beneath.

Locate the centre of the second shape.

Draw a small $x$ in the centre of the square (this does not have to be exact)

2 Use a ruler to visualise a horizontal and/or vertical line of symmetry through the centre of the shape.

Hither we tin can draw a vertical line as this divides the shape into ii identical rectangles (ane is a reflection of the other), and a horizontal line equally this divides the shape into ii coinciding rectangles that are a reflection of each other.

iii Go along to rotate the ruler around $180$ degrees over the eye indicate to cover all sides and vertices.

As the shape has an fifty-fifty number of vertices, nosotros can pass through opposing vertices to see whether there is a line of symmetry present. For the square, we can draw a further ii lines of symmetry (along the diagonals of the square).

The square has $4$ lines of symmetry.

Example ii: using angles (lines of symmetry)

Show that the hexagon beneath has no lines of symmetry.

Locate the center of the 2nd shape.

Depict a small $x$ in the centre of the hexagon (this does not have to be verbal)

Use a ruler to visualise a horizontal and/or vertical line of symmetry through the middle of the shape.

Imagine drawing a line from A to D. If this is a line of symmetry then the angles B and F, and C and E must be equal to one another. Every bit C and Due east are non equal to each other, the line AD is not a line of symmetry.

Proceed to rotate the ruler around $180$ degrees over the center point to cover all sides and vertices.

Equally the shape has an fifty-fifty number of vertices, we need to laissez passer through opposite vertices and then pass through opposing sides. When nosotros pass through opposing vertices, we get:

When nosotros laissez passer through opposing sides, we get:

As there is no line that can be drawn through opposing vertices or sides of the shape that splits the shape into ii reflected parts, this shape has no lines of symmetry.

Example iii: quadratic graph (lines of symmetry)

Write the equation of the line of symmetry for the quadratic equation $y=x^two-8x+12$ .

Locate the centre of the 2D shape.

Here, rather than locating the eye, we are going to locate the turning bespeak of the curve. The turning signal is the indicate on the curve where the slope changes from positive to negative, or vice versa.

For the graph of $y=x^ii-8x+12$ , we can see that the turning bespeak is at $(4,-four)$ however for questions without a visual representation, the turning point can exist calculated by completing the square:

$\begin{aligned} &y=(x-4)^2-16+12\\\\ &y=(10-four)^2-iv \cease{aligned}$

Turning Point: $(4,-4)$ .

Employ a ruler to visualise a horizontal and/or vertical line of symmetry through the middle of the shape.

Here we can run across that if nosotros sketch a vertical line through the turning indicate $(iv,-4)$ , the coordinates on either side of this line are the same distance away from the line. This means that the vertical line that intersects the $x$ axis at $four$ is a line of symmetry, or $x=4$ .

Continue to rotate the ruler around $180$ degrees over the center point to embrace all sides and vertices.

By rotating the ruler around the turning signal, there are no other lines that bear witness a symmetry in the graph of $y=x^2-8x+12$ . The only line of symmetry therefore is $10=four$ .

Case four: the regular hexagon (rotational symmetry)

Calculate the order of rotational symmetry for a regular pentagon.

Locate the middle of the 2D shape.

Draw a small $x$ in the centre of the hexagon (join the opposing vertices together to locate the center)

Trace the shape onto a piece of tracing paper including the middle and northline .

Equally the regular pentagon has a lot of vertices, it is useful to too depict a dot in one vertex so you don't lose sight of what the original looks like:

Rotate the tracing around the center and count the number of identical occurrences .

Rotating the shape around the centre, there are multiple occasions when the shape is identical to the original. Below nosotros accept shown multiple stages of the rotation:

By placing a dot in each position when the shape is identical, we tin can count the gild of rotation once the shape has been rotated $360^o$ around the eye.

The regular pentagon has a rotational symmetry of order $v$ .

Top Tip: A shape cannot have a rotational symmetry of $1$ as we include the original orientation if the shape has a rotational symmetry.

Example v: bending facts (rotational symmetry)

The rhombus ABCD has ii pairs of parallel sides. Use bending facts to calculate the order of rotation for the shape ABCD.

Locate the centre of the second shape.

To find the center of the shape, bring together the diagonals together. Here we have:

Next we need to calculate all of the interior angles of the shape and utilise them to summate the order of rotation:

BCD $= 180-62 = 118^o$ (angles on a straight line total $180^o$ )

ABC $= 62^o$ (alternating angles are equal)

BAD $= 180-62 = 118^o$ (co-interior angles full $180^o$ )

ADC $= 180-118 = 62^o$ (co-interior angles total $180^o$ ).

Nosotros now have the following data:

Trace the shape onto a piece of tracing paper including the centre and northline .

Again, we are going to attempt visualising the rotation without tracing paper by rotating the polygon $360^o$ effectually its centre.

Rotate the tracing around the center and count the number of identical occurrences .

There are 2 occurrences when the paradigm matches the original and so the social club of rotation for the rhombus ABCD is $2$ .

Case 6: cubic graph (rotational symmetry)

Calculate the society of rotational symmetry for the cubic graph $y=x^iii+2$ around the eye $(0,0)$ .

Locate the centre of the 2D shape.

We know the center $(0,0)$ and then let u.s. draw it onto the graph:

Trace the shape onto a piece of tracing paper including the centre and northline .

As the shape is at present a graph, sketch the graph onto a slice of tracing paper. You do non need to include the axes as information technology is the graph that is important.

Rotate the tracing around the centre and count the number of identical occurrences.

If we plow the tracing $180^o$ around the point $(0,0)$ we get a match with the original. This is the only occurrence along with the original and and so the order of rotation for the cubic graph $y=-0.1x^3$ around the betoken $(0,0)$ is $2$ .

Common misconceptions

Rotational symmetry/lines of symmetry

Lines of symmetry are mixed up with rotational symmetry. A line of symmetry divides the shape every bit into 2 symmetrical pieces. A rotational symmetry is the number of times a shape fits into itself when rotated effectually its centre.

The diagonals of quadrilaterals

The diagonals of a quadrilateral are joined together and highlighted every bit a line of symmetry. The only quadrilateral where this is true is the square. Below is the example for an wrong line of symmetry for a rectangle.

The number of sides = the number of lines of symmetry

Although this is true for regular shapes, this is non true for all shapes.

Rotational symmetry of order $one$

A shape cannot have an social club of rotational symmetry of $1$ . This is because the original is included if the shape has a rotational symmetry and and then we must ever accept a rotational symmetry of at least $2$ . If a shape does not accept a rotational symmetry, it is said to have an order of $0$ .

The number of sides = the lodge of rotational symmetry

Although this is true for regular shapes, this is not true for all shapes.

Practice lines of symmetry questions

GCSE Quiz False

GCSE Quiz True

GCSE Quiz False

Practice lines of symmetry question 6 explanation 1

When rotated $180^o$ , the image is the aforementioned as the original.

Symmetry GCSE questions

i. Consummate the table to state the order of rotation for the following shapes

Shape Guild of Rotation

Square

Isosceles Triangle

Parallelogram

Regular Octagon

(4 marks)

Evidence answer

Shape Order of Rotation

Square $\hspace{3cm} iv$

Isosceles Triangle $\hspace{1.6cm} 0$

Parallelogram $\hspace{two.1cm} 2$

Regular Octagon $\hspace{ane.7cm} 8$

(4)

2. (a) Complete table of values for the cubic $y=10^3.$

$\brainstorm{aligned} &ten \quad \quad -ii \quad \quad -1 \quad \quad \quad 0 \quad \quad \quad 1 \quad \quad \quad 2 \quad \quad \quad three \\ &y \quad \quad \quad \quad \quad \;\; -1 \quad \quad \quad 0 \quad \quad \quad 1 \end{aligned}$

(b) James says "the graph of $y=x^3$ has a line of symmetry at $x=0.$ " Is he correct? Explain your answer.

(half dozen marks)

Show answer

(a)

$\begin{aligned} &ten \quad \quad -two \quad \quad -1 \quad \quad \quad 0 \quad \quad \quad 1 \quad \quad \quad 2 \quad \quad \quad iii \\ &y \quad \quad -8 \quad \quad -1 \quad \quad \quad 0 \quad \quad \quad 1 \quad \quad \quad 8 \quad \quad \quad 27 \end{aligned}$

(i)

(b)

(one)

$y$ is positive when $x>0$ and y is negative when $ten<0$ then they are non symmetrical

(1)

(c)

$ii$

(1)

iii. Calculate the equation of the line of symmetry for the line $y=4x+one$ at the indicate $(4,17).$

(3 marks)

Show respond

Perpendicular slope $chiliad=-0.25$

(one)

$y=-0.25x+c$ at $(ii,9)$ means $17=-0.25 \times 4+c, \; c=eighteen$

(1)

$y=-0.25x+18$

(one)

Learning checklist

You have now learned how to:

Identify lines of symmetry in 2-D shapes presented in different orientations

Even so stuck?

Prepare your KS4 students for maths GCSEs success with Third Infinite Learning. Weekly online ane to one GCSE maths revision lessons delivered past expert maths tutors.

Notice out more nearly our GCSE maths revision programme.

NULL

We employ essential and non-essential cookies to improve the experience on our website. Please read our Cookies Policy for information on how we use cookies and how to manage or change your cookie settings.Accept